Funksioni drejtkëndor

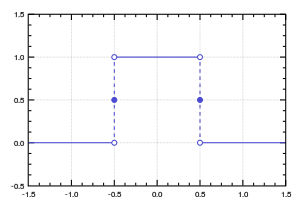
Funksioni drejtkëndor (i njohur gjithashtu si funksioni i drejtë, funksioni i portës ^[1]ose pulsi i njësisë) përcaktohet në mënyrë të tillë që:

$\operatorname {rect} \left({\frac {t}{a}}\right)=\Pi \left({\frac {t}{a}}\right)=\left\{{\begin{array}{rl}0,&{\text{if }}|t|>{\frac {a}{2}}\\{\frac {1}{2}},&{\text{if }}|t|={\frac {a}{2}}\\1,&{\text{if }}|t|<{\frac {a}{2}}.\end{array}}\right.$

Përkufizimet alternative të funksionit përcaktojnë ${\textstyle \operatorname {rect} \left(\pm {\frac {1}{2}}\right)}$ të jetë 0, ^[2] 1, ^[3] ^[4] ose e papërcaktuar.

Versioni i tij periodik quhet valë drejtkëndore .

Transformimi Furier i funksionit drejtkëndor

Transformimet unitare të Furierit të funksionit drejtkëndor janë $\int _{-\infty }^{\infty }\operatorname {rect} (t)\cdot e^{-i2\pi ft}\,dt={\frac {\sin(\pi f)}{\pi f}}=\operatorname {sinc} _{\pi }(f),$ duke përdorur frekuencën e zakonshme f, ku <math xmlns="http://www.w3.org/1998/Math/MathML"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <msub> <mi>sinc</mi> <mrow class="MJX-TeXAtom-ORD"> <mi>π</mi> </mrow> </msub> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle \operatorname {sinc} _{\pi }}</annotation> </semantics> </math><img alt="{\displaystyle \operatorname {sinc} _{\pi }}" aria-hidden="true" class="mwe-math-fallback-image-inline mw-invert skin-invert" data-cx="{"adapted":false}" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/02e16b853b0c49d919aa5088d75df5dc9386c2f9" style="vertical-align: -0.671ex; width:5.062ex; height:2.509ex;"> është forma e normalizuar ^[5] e funksionit sinc dhe ${\frac {1}{\sqrt {2\pi }}}\int _{-\infty }^{\infty }\operatorname {rect} (t)\cdot e^{-i\omega t}\,dt={\frac {1}{\sqrt {2\pi }}}\cdot {\frac {\sin \left(\omega /2\right)}{\omega /2}}={\frac {1}{\sqrt {2\pi }}}\operatorname {sinc} \left(\omega /2\right),$ duke përdorur frekuencën këndore $\omega$ , ku <math xmlns="http://www.w3.org/1998/Math/MathML"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>sinc</mi> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle \operatorname {sinc} }</annotation> </semantics> </math><img alt="{\displaystyle \operatorname {sinc} }" aria-hidden="true" class="mwe-math-fallback-image-inline mw-invert skin-invert" data-cx="{"adapted":false}" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/876707323d2d558232894c2c9e776a93a1206ade" style="vertical-align: -0.338ex; width:3.888ex; height:2.176ex;"> është forma e panormalizuar e funksionit sinc .

Për $\operatorname {rect} (x/a)$ , TF i tij është $\int _{-\infty }^{\infty }\operatorname {rect} \left({\frac {t}{a}}\right)\cdot e^{-i2\pi ft}\,dt=a{\frac {\sin(\pi af)}{\pi af}}=a\ \operatorname {sinc} _{\pi }{(af)}.$ Vini re se për sa kohë që përkufizimi i funksionit të pulsit motivohet vetëm nga sjellja e tij në përvojën e rrafshit të kohës, nuk ka asnjë arsye për të besuar se funksioni i TF duhet të jetë intuitiv, ose i kuptuar drejtpërdrejt nga njerëzit . Megjithatë, disa aspekte të rezultatit teorik mund të kuptohen në mënyrë intuitive, pasi jopafundësia në rrafshin e kohës përkon me një përgjigje të frekuencës së pafundme. (E anasjelltas, një transformim i fundmë Furier do t'i korrespondojë përgjigjes së domenit kohor të pafund)

Përdorimi në probabilitetit

Duke parë funksionin drejtkëndor si FDP, është një rast i veçantë i shpërndarjes uniforme të vazhdueshme me $a=-1/2,b=1/2.$ Funksioni karakteristik është

$\varphi (k)={\frac {\sin(k/2)}{k/2}},$

dhe funksioni i tij gjenerues i momentit është

$M(k)={\frac {\sinh(k/2)}{k/2}},$

ku $\sinh(t)$ është funksioni i sinusit hiperbolik .

Funksioni delta i Dirakut

Funksioni drejtkëndësh mund të përdoret për të përfaqësuar funksionin delta të Dirac $\delta (x)$ . ^[6] Konkretisht, $\delta (x)=\lim _{a\to 0}{\frac {1}{a}}\operatorname {rect} \left({\frac {x}{a}}\right).$

^ Wolfram Research (2008). "HeavisidePi, Wolfram Language function". Marrë më 11 tetor 2022. {{cite web}}: Mungon ose është bosh parametri |language= (Ndihmë!)
^ Wang, Ruye (2012). Introduction to Orthogonal Transforms: With Applications in Data Processing and Analysis. Cambridge University Press. fq. 135–136. ISBN 9780521516884. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)
^ Tang, K. T. (2007). Mathematical Methods for Engineers and Scientists: Fourier analysis, partial differential equations and variational models. Springer. fq. 85. ISBN 9783540446958. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)
^ Kumar, A. Anand (2011). Signals and Systems. PHI Learning Pvt. Ltd. fq. 258–260. ISBN 9788120343108. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)
^ Wolfram MathWorld, https://mathworld.wolfram.com/SincFunction.html
^ Khare, Kedar; Butola, Mansi; Rajora, Sunaina (2023). "Chapter 2.4 Sampling by Averaging, Distributions and Delta Function". Fourier Optics and Computational Imaging (bot. 2nd). Springer. fq. 15–16. doi:10.1007/978-3-031-18353-9. ISBN 978-3-031-18353-9. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)

[1] Wolfram Research (2008). "HeavisidePi, Wolfram Language function". Marrë më 11 tetor 2022. {{cite web}}: Mungon ose është bosh parametri |language= (Ndihmë!)

[2] Wang, Ruye (2012). Introduction to Orthogonal Transforms: With Applications in Data Processing and Analysis. Cambridge University Press. fq. 135–136. ISBN 9780521516884. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)

[3] Tang, K. T. (2007). Mathematical Methods for Engineers and Scientists: Fourier analysis, partial differential equations and variational models. Springer. fq. 85. ISBN 9783540446958. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)

[4] Kumar, A. Anand (2011). Signals and Systems. PHI Learning Pvt. Ltd. fq. 258–260. ISBN 9788120343108. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)

[5] Wolfram MathWorld, https://mathworld.wolfram.com/SincFunction.html

[:0-6] Khare, Kedar; Butola, Mansi; Rajora, Sunaina (2023). "Chapter 2.4 Sampling by Averaging, Distributions and Delta Function". Fourier Optics and Computational Imaging (bot. 2nd). Springer. fq. 15–16. doi:10.1007/978-3-031-18353-9. ISBN 978-3-031-18353-9. {{cite book}}: Mungon ose është bosh parametri |language= (Ndihmë!)

[1]

[2]

[3]

[4]

[5]

[6]